Introduction
In this document we'll describe a method for measuring the line width of single longitudinal mode lasers. Such lasers have very narrow (few MHz) spectral line widths, long coherence length, and very low phase noise.
A common figure of merit for an optical spectrometer quantifies its ability to distinguish between two adjacent wavelengths, \( \delta \lambda = \lambda_1 - \lambda_2\) or frequencies \( \delta \nu = \nu_1 - \nu_2\). Spectrometers encountered in a physics lab, based on prisms or diffraction gratings, typically have spectral resolving power, \(\lambda /\delta \lambda = \nu /\delta\nu\) , of a few hundred to a few tens of thousands. To achieve higher resolving power new methods, typically based on interferometers, are employed.
The heterodyne spectrometer described here achieves a spectral resolving power of almost \(10^9\). We use a Mach-Zehnder interferometer coupled with a heterodyne method to measure the spectral line shape and width of radiation emitted by a laser diode, as developed by T. Okoshi et al. (1980 "Novel method for high resolution measurement of laser output spectrum," Electron. Lett. 16, 630).
In the first section we review the hardware configuration. In the second section we review how to deduce the linewidth from the interferometer signal. A video tutorial on the Eikonal Optics web page shows the spectrometer hardware, presents experimental results, and illustrates real time fitting the laser line width using the theory developed below.
Experimental Setup and Measurements
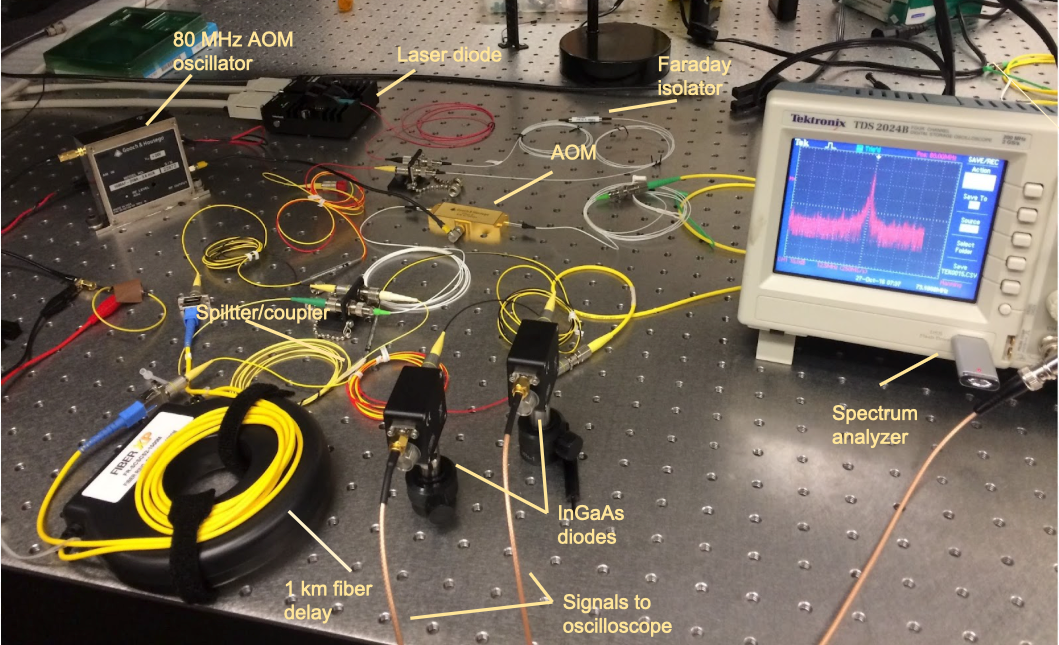
The light source (Fig. 1) examined here is a 1550 nm (193 THz) distributed feedback (DFB) laser diode. DFB diodes are one of the core technologies in dense wavelength division multiplexing (DWDM) fiber telecom systems and are characterized by very narrow linewidths (several MHz or less). The need for narrow linewidth comes from the requirement that DWDM systems multiplex up to 160 communication channels in a single SMF-28e fiber using 25 GHz channel spacings.

Figure 2 shows the experimental configuration where light from the diode is split into two paths with one injected into a Bragg cell also known as an acousto-optic modulator (AOM). In an AOM a piezoelectric actuator is energized by a radio frequency (80 MHz) signal, which launches an acoustic wave into a transparent optical medium. Photons interact with the acoustic phonons in a manner consistent with the Bragg condition for constructive interference (see Appendix A). To conserve energy and momentum the scattered photons are Doppler shifted in frequency by an amount equal to the phonon frequency. When light directly from the laser diode is recombined with that which passed through the AOM the two waves interfere, yielding beats at a frequency, \(\Omega\), equal to that of the RF signal driving the AOM. If the two waves are mixed on a fast photodiode then an electrical RF signal can be detected. This RF signal encodes information about the intrinsic line shape emitted by the laser diode.
In the absence of a delay between the two paths, the signals reaching the photodiode will be perfectly correlated and the diode will record a signal that is a delta-function at frequency \(\Omega\). However, if a sufficiently long time-delay is introduced, the two signals are temporally decorrelated. In this circumstance, any frequency fluctuations that are present in the original source will now be evident as a broadening of the beat frequency signal.

Figure 3 shows the physical set up. The experiment uses two fiber splitters-one to divide the light and one to recombine it. A splitter is the fiber equivalent of a free-space beam splitter or a partially reflecting mirror. A splitter is formed by stripping the cladding from a length of two fibers and wrapping the two resultant cores together. A bond is formed between the cores by tensioning the fibers and heating the junction until the two fibers are tapered and fused. Such a device is known as a fused biconical taper (FBT) splitter. This configuration can be used either as a splitter or as a combiner.

Unlike the sketch in Fig. 2, each splitter has two inputs and two outputs. Only one of the inputs is used for the input splitter; both outputs of the combiner are used to feed two photodiodes, although only one is needed for this application. These splitters/combiners are bidirectional, allowing any port to be used as an input.
A Faraday isolator is included between the laser diode and the interferometer. This prevents reflections from returning to the laser and modifying the conditions in the gain medium, which might cause fluctuations in intensity or frequency. An isolator consists of two linear polarizers separated by a Faraday rotator, which rotates the plane of polarization by 45\(^\circ\). Any reflections suffer a second pass through the Faraday rotator and an additional 45\(^\circ\) rotation so that the plane of polarization is now perpendicular to the original linear polarizer. Consequently, reflected light is suppressed, typically by 30 dB.

A Sketch of Heterodyne Theory
Intrinsic Laser Line Shape
The theory of the self-heterodyne method can be found in Richter et al. (1986 IEEE J Quant. Elect. 22, 2070). Here we add a few additional steps to help clarify the derivation and highlight the assumptions adopted.
First we develop a characterization of the spectral line shape \( S_E ( \omega ) \) produced by the laser. Suppose the resultant electric field generated by the laser is described by
\[ E(t) = E_0 \exp \{ \mathbb{i} \left[ \omega_o t + \phi(t) \right] \tag{1} \} , \]where departures from pure monochromatic behavior at laser angular frequency \( \omega_0 \) are characterized by time dependent random variations in \( \phi (t), \) known as phase jitter. Fluctuations exist in both amplitude and phase. In each round-trip in the laser cavity, some noise amplitude is added to the electric field. This changes both amplitude and phase of the field. However, because of gain saturation there is feedback which drives the power to the steady-state value and hence fluctuations in \( E_0 \) are damped. In contrast, there is no phase feedback, which therefore exhibits a random walk, leading to a finite emitted line width. Consequently, it is safe to ignore fluctuations in the amplitude of the electric field \( E_0 \).
We find the laser line shape by first evaluating the autocorrelation function associated with the fluctuations in \( \phi \) and then using the Wiener-Khinchin theorem to compute the associated spectrum, \( S_E (\omega ) \).
The autocorrelation function is defined as the expectation value \[ \Gamma(\tau) = \langle E^*(t) E(t + \tau ) \rangle, \tag{2} \] or in terms of the random phase change between times \(t \) and \( t + \tau \), \[ \Delta \phi(t,\tau ) = \phi(t+\tau) - \phi(t) . \] We assume that the fluctuations \(\Delta \phi(t,\tau) \) follow a Gaussian distribution with zero mean and variance given by \[ \sigma^2_\phi ( \tau ) = \langle \left[ \phi(t+\tau) - \phi(t) \right]^2\rangle . \] The phase error variance therefore follows a random walk that grows linearly with time, in which case the phase variance is conveniently written as \[ \sigma^2_\phi (\tau) = \tau/\tau_c , \] where the constant or proportionality \(\tau_c\) is called the coherence time.
Evaluating the autocorrelation function, Eq. (2) using Eq. (1) we find \[ \Gamma(\tau) =\langle \exp \left[ \mathbb{i} \Delta \phi(t,\tau ) \right] \rangle \exp(\mathbb{i} \omega_0 \tau) , \]
and the expectation value integral simplifies to \[ \Gamma(\tau) = \exp \left[ - \sigma^2_\phi (\tau )/2 \right] \exp(\mathbb{i} \omega_0 \tau) ,\]
using the Gaussian moment theorem \(\langle \exp (\mathbb{i} x ) \rangle = \exp ( -\langle x^2 \rangle/2 ) \) for a random variable \( x \) with zero mean.
Using the Wiener-Khinchin theorem, the Fourier transform of \( \Gamma (\tau) \) yields the line shape power spectral density \( S_E (\omega) \) \[ S_E (\omega) = \frac{E_0^2 }{\pi } \frac{ \gamma }{\gamma^2 + (\omega-\omega_0)^2} , \] where \( 2 \gamma = 1/\tau_c \) is the angular frequency full line width at half maximum of the resultant Lorentzian profile.
We now see that the constant \(2 \gamma \) is the laser line width given by the famous Schawlow-Townes (1958 Phys. Rev. 112 1940) formula in terms of the natural atomic line width and properties of the laser cavity.
Heterodyne Case
We now describe the situation in this experimental configuration where the light from the laser is split and recombined.
The total electric field incident on the detector \[ E_T(t) = E_0 \exp\{\mathbb{i} [ \omega_0 t + \phi(t) ]\} + E_0 \exp\{\mathbb{i} [ (\omega_0 t + \Omega)(t-\tau_0) + \phi(t-\tau_0)] \}, \]
is the sum of the electric field from each arm of the interferometer. The quantity \( \tau_0\) is the differential time delay of the two interferometer paths,and \( \Omega \) is the frequency shift introduced by the acousto-optic modulator. We made the assumption that the electric field has unit amplitude in both arms of the interferometer.
In our experiment a photodiode is used to convert the phase fluctuations of \( E_T(t) \) into intensity noise and hence a fluctuating photocurrent which is recorded by the oscilloscope. Photocurrent is linearly proportional to the incident power which in turn is proportional to \( E_T^*(t) E_T(t)\). Therefore, the autocorrelation function for the photocurrent is \[ \Gamma_T (\tau) = \langle E_T^*(t) E_T (t) E_T^*(t+\tau) E_T (t+\tau) \rangle . \]
Substitution of the expression for \( E_T(t) \) and multiplying out yields 16 terms, ten of which average to zero because of the \( \exp (\mathbb{i} \Omega t ) \) oscillation. The remaining terms simplify to \[ \Gamma_T(\tau) = E_0^4 \left[ 4 + 2 \cos (\Omega \tau) \exp\left( -\sigma_\phi^2 (\tau_0) - \sigma_\phi^2 (\tau) + \sigma_\phi^2 (\tau+\tau_0)/2 + \sigma_\phi^2 (\tau-\tau_0)/2 \right) \right] , \]
again making use of the Gaussian moment theorem.
Ignoring the delta function at zero frequency associated with photon shot noise, the spectrum of photocurrent fluctuations is given by Fourier transformation to yield \[\begin{eqnarray} S_T(\omega) & = & \frac{1}{\pi} \frac{2\gamma }{(2\gamma)^2+(\omega-\Omega)^2} \left\{1-\left[\cos(\omega-\Omega)\tau_0+\frac{2\gamma \sin (\omega-\Omega)\tau_0}{(\omega-\Omega)}\right] \exp(- 2\gamma\tau_0 ) \right\}\ + \\ & & \\ & & \delta(\omega - \Omega) \exp(-2\gamma\tau_0) . \tag{3} \end{eqnarray}\]
The measured RF power is proportional \(E_0^4\). We have dropped the normalizing factor in Eq. (3) because we have not determined the coupling efficiency of the diode to the interferometer or calibrated the photodiode responsivity.
According to Eq. (3) as \( \tau_0 \) increases in relation to \(\tau_c\) the signal shifts from a delta function to a modified Lorentzian with interference ripples until the power spectrum becomes strictly Lorentzian when the delay is much longer than the coherence time.
Figure 5 plots some examples of the behavior predicted by Eq. (3). In this example the delta function at \( \omega = \Omega\) has been suppressed and the spectra are normalized to unity. The figure shows that in the limit of large delay times, \( \tau_0\), when the phase of the two optical fields at the photodiode have become decorrelated, the noise power spectrum becomes exactly Lorentzian with width \( 4 \gamma \), or twice the intrinsic laser linewidth. Figure 5 also shows the effect on the measured noise spectrum of finite \( \tau_0 \) (in units of the coherence time, \(\tau_c = 1/(2\gamma ) \)). For delay times which are comparable to or shorter than the coherence time, the quasi-Lorentzian part is broadened and scalloped.
The convolution in Eq. (3) originates from the multiplication of the time-varying local oscillator field with the signal field in the photodetector. Multiplication in the time-domain is equivalent to convolution in the frequency domain. Hence the measured power spectrum has a width twice that of the original line.

If you would like Eikonal to characterize a laser diode for you or design a spectrometer to measure your laser diode properties, please contact us.
Appendix A: Acousto-Optic Modulators
A Bragg cell or acousto-optic modulator (AOM) is a device which controls light using an electrical signal. Typically, an AOM comprises a crystalline or glass optical medium excited by a piezoelectric transducer. The compressions and rarefactions due to the associated acoustic wave induce periodic, spatial index of refraction variations (the acousto-optic effect), which cause the incident beam to diffract. When the interaction occurs over an extended volume, light can experience Bragg diffraction; accordingly, AOMs are sometimes called Bragg cells.
where \( n \) is the index of refraction, \(\Lambda\) is the wavelength of the acoustic wave and \(\lambda \) is the optical wavelength. For typical parameters, angular deflections (2\(\theta\)) of a few degrees are practical given acoustic excitation at high frequencies (~ 10-100 MHz).
Since the acoustic wave is slow compared to the light wave ( \( v_s / c \simeq 10^{-5}\)), the acousto-optic perturbation can be thought of as a stationary volume grating. However, because the acoustic wave is moving, the light also experiences a Doppler shift in frequency according to \[ \Delta \nu /\nu = 2 n \nu \sin \theta / c ,\]
where \( v_s \sin \theta\) is the component of the acoustic wave speed in the direction of the incident beam. When the Bragg condition is satisfied the frequency shift is \(\Delta \nu = v_s /\Lambda \), or equal to the frequency of the acoustic wave.
Alternatively, suppose a photon with energy \( h \nu\) and momentum \( \hbar \vec{k} \) absorbs an acoustic phonon with energy \( h V \) and momentum \( \hbar \vec{K}\). Conservation of energy and momentum requires \( \nu' = \nu + V\) and \( \vec{k'} =\vec{k}+\vec{K}\). As optical frequencies (THz) are much greater then acoustic frequencies (MHz) \( k \gg K\), and this requires that \(2 k \sin \theta = K\). Furthermore, when \( k \gg K\) the small angle approximation yields the scattering angle \(2\theta \simeq K/k\). In the current example \( K/k\) = 80 MHz/193 THz = \( 4.2\times 10^{-7}\).

In summary the optical frequency of the scattered beam is increased or decreased by the frequency of the sound wave (depending on the propagation direction of the acoustic wave relative to the beam) and propagates in a slightly different direction.
© Eikonal Optics